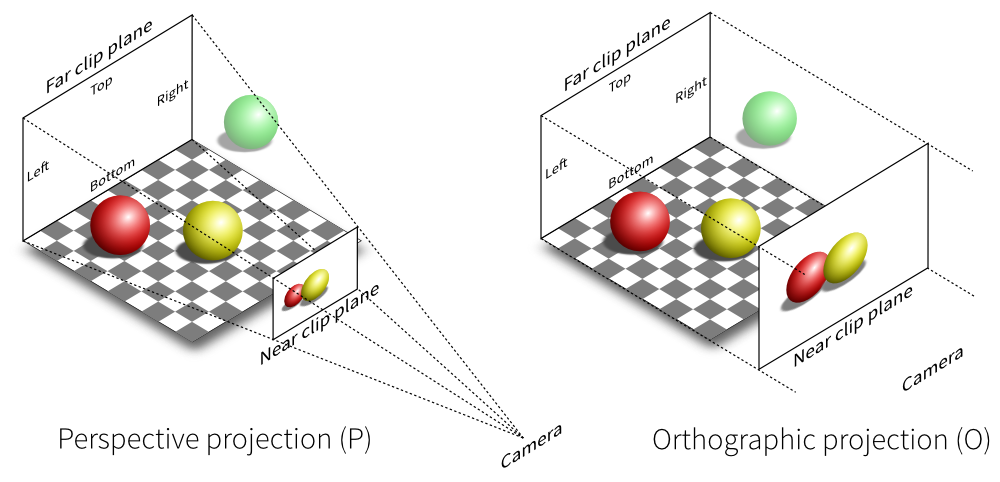
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
84
85
86
87
88
89
90
| #include <bits/stdc++.h>
using namespace std;
const int iol = 1024 * 1024;
char buf[iol], *ioh, *iot, ioc;
bool iosig;
inline char read() {
if (ioh == iot) {
iot = (ioh = buf) + fread(buf, 1, iol, stdin);
if (ioh == iot) return -1;
}
return *ioh++;
}
template<class T>
inline bool read(T& x) {
iosig = false;
for (ioc = read(); !isdigit(ioc); ioc = read()) {
if (ioc == -1) return false;
if (ioc == '-') iosig = true;
}
x = 0;
while (ioc == '0') ioc = read();
for (; isdigit(ioc); ioc = read())
x = (x << 1) + (x << 3) + (ioc ^ '0');
ioh--;
if (iosig) x = -x;
return true;
}
const int MAXN = 100010;
int n, tot;
int father[MAXN], son[MAXN * 20][2], pre[MAXN * 20], dis[MAXN];
struct Node {
int v, w;
Node() {}
Node(int v, int w) : v(v), w(w) {}
};
std::vector<Node> edge[MAXN];
inline void addEdge(int u, int v, int w) {
edge[u].push_back(Node(v, w));
edge[v].push_back(Node(u, w));
}
inline void bfs(int u = 1) {
queue<int> q;
q.push(u);
while (!q.empty()) {
int x = q.front();
q.pop();
for (int i = 0; i < edge[x].size(); i++) {
Node *now = &edge[x][i];
if (now->v != father[x]) {
father[now->v] = x;
dis[now->v] = dis[x] ^ now->w;
q.push(now->v);
}
}
}
}
int main() {
int maxw = 0;
read(n);
for (int i = 0, u, v, w; i < n; i++)
read(u), read(v), read(w), addEdge(u, v, w), maxw = max(maxw, w);
bfs();
int log = log2(maxw);
for (int i = 1, tmp; i <= n; i++) {
tmp = 0;
for (int j = log, x; j >= 0; j--) {
x = (dis[i] & (1 << j)) ? 1 : 0;
if (!son[tmp][x]) son[tmp][x] = ++tot;
tmp = son[tmp][x];
}
pre[tmp] = i;
}
int ans = 0;
for (int i = 1, tmp; i <= n; i++) {
tmp = 0;
for (int j = log, x; j >= 0; j--) {
x = (dis[i] & (1 << j)) ? 1 : 0;
if (son[tmp][!x]) tmp = son[tmp][!x];
else tmp = son[tmp][x];
}
ans = max(ans, dis[i] ^ dis[pre[tmp]]);
}
cout << ans;
return 0;
}
|